The Universe is Expanding
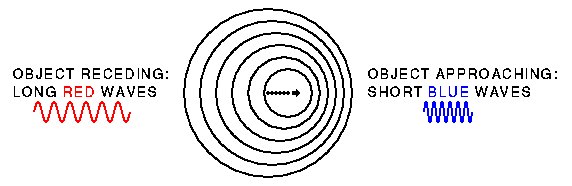
Doppler Effect
Without going into the math, we will simply note that the redshift (z) is measured by taking the change in the wavelength and dividing it by the normal wavelength.
z = change in wavelength / normal wavelength
What is truly remarkable is that the redshift (z) is also the proportion of the velocity (v) of recession to the speed of light (c). So to find the velocity v of recession of a galaxy, we need only multiply z by the speed of light c.
z = v / c
which is the same as
v = z c
As we will see later, the speed of light is always the same. No matter how we make the measurement, we will find light moving at about 300,000 km/s. Multiply z by this speed, and you'll have that the velocity at which the galaxy is moving away from us.
Example: For the supercluster of galaxies known as BAS11, the redshift (the change in the wavelength divided by the normal wavelength) was measured to be a tiny change of z = 0.07. Since v = z c then 0.07 = v / c, and the velocity of recession of the cluster of galaxies is a huge v = 0.07 c or 21,000 km/s .
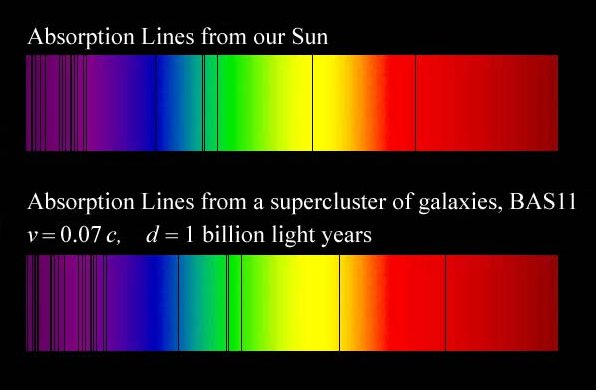
Redshift for Supercluster of Galaxies BAS11
The pattern of the spectral lines remains the same, but their wavelengths are all increased, and the lines shift to the red.
Edwin Hubble compared redshifts with the distances of the nearby galaxies he could measure at the time, and he found that the farther the galaxy from us, the faster it was receding away. Today, using the inverse square law with Type Ia supernovae, the effect has been studied with high precision. We find that galaxies recede about 70 km/sec faster for each megaparsec more distant they are. This is written 70 km/s / Mpc. (Recall that a parsec is 3.26163 light years. "Mega " means a million, so 1 megaparsec is 3,261,630 light years. Megaparsecs are used to keep the math simple.)
This 70 km/s / Mpc is called the Hubble Constant, and is written simply as H. There is some uncertainty on the exact value of H, but about 71 km/s / Mpc is the best measurement we have to date. We use H = 70 km/s / Mpc to keep things simple.
Hubble Law
Here it is at last! The great Hubble's law used to measure the universe!
v = H D
Example: For the supercluster of galaxies BAS11, we have already measured the velocity v of recession for the galaxy cluster to be 21,000 km/s. We use H = 70 km/s / Mpc, to find the distance in megaparsecs (Mpc). For BAS11 we get
v = H D
21000 km/s = 70 km/s / Mpc x D
300 Mpc = D
The BAS11 supercluster is about 300 Mpc distant, or 978,489,000 light years. That's roughly a billion light years.
Comparing Distances of Galaxies with Hubble's Law
Below is a graph showing measured velocities and distances to galaxies compared to Hubble's Law. Each point on ths graph is the average distance and speed of a cluster of hundreds of galaxies. The Virgo Cluster is the closest one to us. Notice that not only is the universe expanding, but the more distant the galaxies the faster they are receding from us!
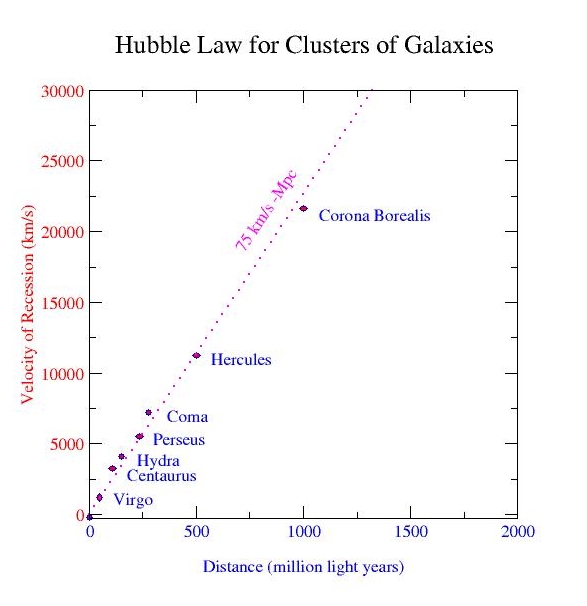
Examples of Hubble's Law

The More Distant the Galaxy, the Gaster it is Receding
In all directions the faster a galaxy is receding the more distant it is. Here is a map of galaxy distances and distributions. Galaxies group in clusters and filaments everywhere.
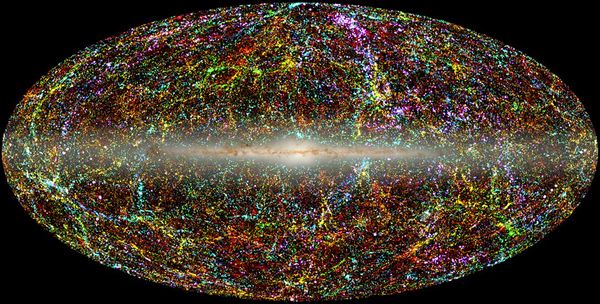
Panorama of 1.5 million galaxies as they appear on the sky, color coded by "redshift." Blue galaxies are the nearest sources (z less than 0.01); green are at moderate distances (z from 0.01 to 0.04) and red are the most distant ones seen in a survey using infrared light that covers z out to 0.1. Credit: T. Jarrett and the 2MASS Redshift Catalog
We have looked at relatively nearby galaxies so far, with fairly small z, but when dealing with galaxies very far away, and receding from us at nearly the speed of light (close to 300,000 km/s ), we must take into account the effects of General Relativity. That will come later, for objects such as the young cluster around the radio galaxy known as TNJ1338-1942, that's at a remarkable z=4.11.
Think for a moment what that means about the wavelengths we would see from this galaxy, and the velocity we would get for it. Eek!
You might explore some of entries on the Wikipedia List of Galaxy Clusters to see the variety in the structure of the universe around us. The Sloan Survey is currently mapping the Universe in 3D by using the Hubble Law to find the distances to galaxies.